Parabolic dynamics
1. Survey
1.1. What does hyperbolic, elliptic mean in dynamics?
Let  be a dynamical system. Even if deterministic, it can exhibit a chaotic behaviour. This has several characteristics. One of them is the Butterfly effect: sensitive dependence on initial conditions (SDIC).
be a dynamical system. Even if deterministic, it can exhibit a chaotic behaviour. This has several characteristics. One of them is the Butterfly effect: sensitive dependence on initial conditions (SDIC).
Definition 1 A flow  has SDIC if there exists
has SDIC if there exists  such that
such that 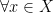 ,
,  ,
,  such that
such that 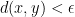 and
and 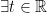 such that
such that 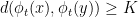 .
.
A quantitative measurement of SDIC is provided by the dependence of  on
on  .
.
Definition 2 Let 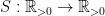 be a nondecreasing function. A flow
be a nondecreasing function. A flow  has SDIC of order
has SDIC of order 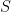 if in the above definition, one can take
if in the above definition, one can take  such that
such that 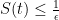 .
.
This leads us to a rough division of dynamical systems:
- Elliptic: no SDIC, or if any, subpolynomial.
- Hyperbolic: SDIC is fast, exponential.
- Parabolic: SDIC is slow, subexponential.
This trichotomy is advertised in Katok-Hasselblatt’s book.
For instance, entropy is a measure of chaos. Elliptic or parabolic dynamical systems have zero entropy. Hyperbolic dynamical systems have positive entropy.
1.2. Examples of elliptic dynamical systems
- Circle diffeomorphisms.
- Linear flows on the torus (these two examples are related, one is the suspension of the other).
- Billiards in convex domains. Usually, there are many periodic orbits, trapping regions, caustics.
This the realm of Hamiltonian dynamics and KAM theory.
1.3. Examples of hyperbolic dynamical systems
- Automorphisms of a torus which are Anosov, i.e. all eigenvalues have absolute values
 . Then orbits diverge exponentially: if
. Then orbits diverge exponentially: if  ,
, 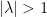 , set
, set  . Then
. Then 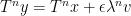 mod
mod 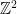 and
and  reaches
reaches  in time
in time  such that
such that  for
for  , an exponential.
, an exponential. - Geodesic flows on constant curvature surfaces.
- Sinai’s billiard: a rectangle with a circular obstacle. Somewhat equivalent to the motion of two hard spheres on a torus. Scattering occurs after hitting the obstacle, due to its strict convexity.
This is the realm of Anosov-Sinai and others’ dynamics. Structural stability occurs: hyperbolic systems form an open set.
1.4. Examples of parabolic dynamical systems
- Horocycle flows on constant curvature surfaces. They were introduced by Hedlund, followed by Dani, Furstenberg, Marcus, Ratner.
- Nilflows on nilmanifolds. If
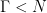 is a cocompact lattive in a nilpotent Lie group, let
is a cocompact lattive in a nilpotent Lie group, let  be a
be a  -parameter subgroup of
-parameter subgroup of  acting by right translations on
acting by right translations on  . It descends to
. It descends to  . Both examples are algebraic, this provides us with tools to study them. They are a bit too special to illustrate parabolic dynamics.
. Both examples are algebraic, this provides us with tools to study them. They are a bit too special to illustrate parabolic dynamics. - Smooth area-preserving flows on higher genus surfaces.
- Ehrenfest’s billiard: rectangular, with a rectangular obstacle. Here, SDIC is only caused by discontinuities due to corners. More generally, billiards in rational polygons (angles belong to
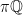 ), or equivalently linear flows on translation surfaces. This field is known as Teichmüller dynamics. Forni considers them as elliptic systems with singularities, I prefer to stress their parabolic character.
), or equivalently linear flows on translation surfaces. This field is known as Teichmüller dynamics. Forni considers them as elliptic systems with singularities, I prefer to stress their parabolic character.
1.5. Uniformity
Within hyperbolic dynamics, there is a subdivision in uniformly hyperbolic, nonuniformly hyperbolic and partially hyperbolic.
In the same manner, we see horocycle flows as uniformly parabolic, and nilflows as partially parabolic, with both elliptic and parabolic directions. Area-preserving flows on surfaces have fixed points which introduce partially parabolic behaviour: shearing is uniform or not. However, there is no formal definition.
1.6. More examples of parabolic behaviours
Parabolicity is not stable. However, Ravotti has discovered a  -parameter perturbation of unipotent flows in
-parameter perturbation of unipotent flows in 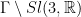 .
.
A flow 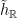 is a time-change of a given flow
is a time-change of a given flow 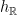 if there exists a function
if there exists a function 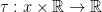 such that
such that
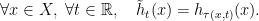
For 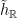 to be a flow, it is necessary that
to be a flow, it is necessary that  be a cocycle.
be a cocycle.
Both flows have the same trajectories. A feature of parabolic dynamics is that a typical time-change 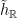 is not isomorphic to
is not isomorphic to 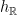 and has new chaotic features. Indeed, an isomorphism would solve the cohomology equation, and there are obstructions.
and has new chaotic features. Indeed, an isomorphism would solve the cohomology equation, and there are obstructions.
1.7. Program
Study smooth time-changes of algebraic flows.
Goes back to Marcus in the 1970’s. Algebraic tools break down, softer methods are required: geometric mechanisms. Also, we expect the features exhibited by time changes to be more typical.
2. Chaotic properties
2.1. Definitions
Definition 3 Let 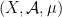 be a measure space with finite measure. Let
be a measure space with finite measure. Let  be a measure preserving flow. The trajectory of a point
be a measure preserving flow. The trajectory of a point  is equidistributed with respect to
is equidistributed with respect to  if for every smooth observable
if for every smooth observable 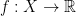 ,
,
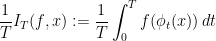 tends to
tends to  as
as 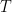 tends to
tends to 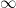 .
.
 is ergodic if
is ergodic if  almost every
almost every  has equidistributed orbit with respect to
has equidistributed orbit with respect to  .
.
This is Boltzmann hypothesis.
Definition 4 Say  is mixing if for all
is mixing if for all  , the correlation
, the correlation
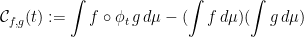 tends to
tends to  as
as  tends to
tends to 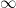 .
.
This means decorrelation of functions. This implies ergodicity.
The speed at which decorrelation occurs is a significative feature too.
Definition 5 The speed of mixing is a function 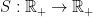 such that for all smooth observables
such that for all smooth observables 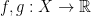 , the correlation decays at speed
, the correlation decays at speed 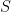 , i.e.
, i.e.
 as
as  tends to
tends to 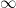 .
.
2.2. Relation to the trichotomy
Elliptic systems often are not ergodic, but even when they are, they are not mixing.
Hyperbolic and parabolic systems can be mixing, but at different speeds:
- hyperbolic
 exponential decay of correlations.
exponential decay of correlations. - in parabolic systems, we expect that, if mixing occurs, the decay of correlations is slower: polynomial or subpolynomial.
A related concept is that of polynomial deviations of ergodic averages: if  is smooth and has vanishing integral,
is smooth and has vanishing integral,  s ergodic and
s ergodic and  is an equidistribution point,
is an equidistribution point,
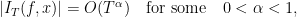
and no faster. This phenomenon was first discovered on horocycle flows, then Teichmüller flows (Zorich, experimentally, Kontsevitch-Zorich for a proof).
2.3. Other features
Spectral properties. Let 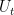 be the operator
be the operator 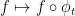 on
on  . Then
. Then 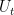 is unitary. What is its spectrum?
is unitary. What is its spectrum?
Disjointness of rescalings. Rescaling means linear time change 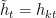 .
.
3. Results
Horocycle flow is mixing (Ratner). The spectrum is Lebesgue absolutely continuous.
Time changes of horocycle flows are mixing (Marcus, by shearing). This can be made quantitative (Forni-Ulcigrai).
Disjointness of rescalings fails for horocycle flows, but hold for nontrivial time changes (Kanigowski-Ulcigrai and Flaminio-Forni). The spectrum is Lebesgue absolutely continuous as well.
Nilflows themselves are not mixing, but typical time changes of nilflows are mixing (Avila-Forni-Ravotti-Ulcigrai).
We shall see geometric mechanisms at work:
- Mixing via shearing.
- Ratner property of shearing.
- Renormizable parabolic flows.
- Deviations of ergodic integrals.
4. Horocycle flows
Today’s goal is to explain the technique of mixing by shearing.
4.1. Algebraic viewpoint
Consider 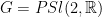 and its subgroups
and its subgroups
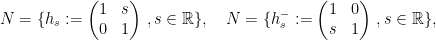

Every matrix can be uniquely written 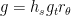 , hence
, hence  . The factors do not commute, because of the key relation
. The factors do not commute, because of the key relation

The key relation can be interpreted as a selfsimilarity property:  is a fixed point of renormalization by
is a fixed point of renormalization by 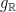 .
.
Take a discrete and cocompact subgroup  . Then
. Then  and
and  act on
act on  by left multiplication. The key relation implies that the rescaled flow $latex {h_{\mathbb R}^k=(h_{ks})_{s\in{\mathbb R}}}&fg=000000$ is conjugated to $latex {h_{\mathbb R}}&fg=000000$. This fails for other (nonrescaling) time changes, as I proved recently with Fraczek and Kanigowski.
by left multiplication. The key relation implies that the rescaled flow $latex {h_{\mathbb R}^k=(h_{ks})_{s\in{\mathbb R}}}&fg=000000$ is conjugated to $latex {h_{\mathbb R}}&fg=000000$. This fails for other (nonrescaling) time changes, as I proved recently with Fraczek and Kanigowski.
4.2. Geometric viewpoint
Let  denote the upper half plane, with metric
denote the upper half plane, with metric 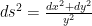 .
.
Fact.  acts isometrically and transitively on
acts isometrically and transitively on  , and this yields a diffeomorphism of
, and this yields a diffeomorphism of  with the unit tangent bundle
with the unit tangent bundle  .
.
Indeed, the action is by Möbius transformations
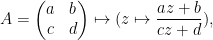
on  , and by their derivatives on
, and by their derivatives on  .
.
With this identification, orbits of  are curves which project to geodesics of
are curves which project to geodesics of  , and coincide with their lifts by their unit speed vector. On the other hand, orbits of
, and coincide with their lifts by their unit speed vector. On the other hand, orbits of  are curves which projects to horocycles of
are curves which projects to horocycles of  , and coincide with their lifts by their unit normal outward pointing vectors. Lifts by inward pointing normal vectors are orbits of
, and coincide with their lifts by their unit normal outward pointing vectors. Lifts by inward pointing normal vectors are orbits of 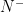 .
.
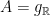 is a hyperbolic flow: it contracts in the direction of
is a hyperbolic flow: it contracts in the direction of 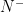 -orbits, it dilates in the direction of
-orbits, it dilates in the direction of  -orbits,
-orbits,
4.3. Classical results
Let  denote Haar measure on
denote Haar measure on  . It maps via
. It maps via  to hyperbolic volume. Consider the induced measure on
to hyperbolic volume. Consider the induced measure on  (still denoted by
(still denoted by  ). Then
). Then  and
and  acts on
acts on  by measure preserving transformations.
by measure preserving transformations.
Then
 is ergodic (Hopf). It is far from being unique ergodic (plenty of periodic orbits).
is ergodic (Hopf). It is far from being unique ergodic (plenty of periodic orbits). is mixing.
is mixing. is uniquely ergodic (Furstenberg). This means that every orbit is equidistributed with respect to
is uniquely ergodic (Furstenberg). This means that every orbit is equidistributed with respect to  .
.
5. Shearing
This is an alternate way to prove mixing. The idea goes back to Marcus (Annals of Math. 1977). Marcus covered a more general situation, and proved mixing of all orders (i.e. for multicorrelations, integrals involving an arbitrarily large number of functions).
Let us shift viewpoint on the key relation. Let 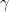 be a piece of
be a piece of  -orbit of length
-orbit of length  . Let
. Let
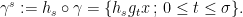
Then  is sheared or tilted in the direction of the geodesic flow.
is sheared or tilted in the direction of the geodesic flow.
Key idea in parabolic dynamics: In several parabolic systems, the Butterfly effect happens in a special way, e.g. shearing. Points nearby move parallel, but with different speeds. This implies that transverse arcs shear.
5.1. Recipe for mixing in parabolic dynamics
Here are the ingredients:
- A uniquely ergodic flow
 .
. - A transverse direction which is sheared in the direction of the flow.
By assumption, for every  , the trajectory
, the trajectory 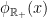 equidistributes with respect to
equidistributes with respect to  . We want to upgrade it to mixing, which is a property of sets: indeed
. We want to upgrade it to mixing, which is a property of sets: indeed
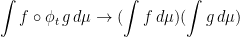
is equivalent to
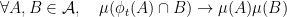
i.e 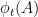 equidistributes as
equidistributes as  .
.
The idea is to cover  by short arcs in the transverse direction. We prove that each such arc equidistributes, and apply Fubini. I.e. if
by short arcs in the transverse direction. We prove that each such arc equidistributes, and apply Fubini. I.e. if  , apply
, apply 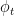 . Then
. Then  .
.
Each  becomes close to a long piece of orbit of
becomes close to a long piece of orbit of  . By unique ergodicity, that piece equidistributes, and this implies equidistribution for
. By unique ergodicity, that piece equidistributes, and this implies equidistribution for  .
.
5.2. Time change
Let 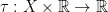 be a smooth time change, which is a cocycle with respect to a given smooth flow
be a smooth time change, which is a cocycle with respect to a given smooth flow 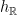 , i.e.
, i.e.
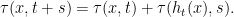
We are interested in the flow  defined by
defined by
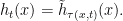
The generator of  is
is
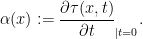
It is a smooth nonnegative function on  . We assume that
. We assume that 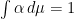 . We denote
. We denote  by
by  .
.
Remark. If 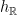 is generated by a smooth vectorfield
is generated by a smooth vectorfield 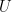 , then
, then 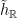 is generated by the vectorfield
is generated by the vectorfield  .
.
Theorem 6 (Forni-Ulcigrai) Let 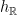 be the horocyclic flow of a compact constant curvature surface. For any smooth function
be the horocyclic flow of a compact constant curvature surface. For any smooth function 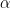 , the flow
, the flow  is mixing, with quantitative estimates which imply that the spectrum is absolutely continuous with respect to Lebesgue measure.
is mixing, with quantitative estimates which imply that the spectrum is absolutely continuous with respect to Lebesgue measure.
Lemma 7 Let  denote the generator of
denote the generator of 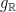 and
and 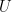 the generator of
the generator of 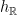 . Take a segment of
. Take a segment of 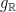 -orbit
-orbit
 Let
Let 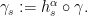 Then
Then 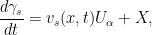 where
where 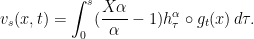
Remark. 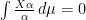 , hence
, hence 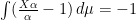 . Thus, as
. Thus, as  tends to
tends to 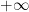 ,
, 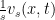 tends to a finite limit, the shear rate.
tends to a finite limit, the shear rate.
Proof of Lemma. It relies on ![{[U,X]=U}](https://s0.wp.com/latex.php?latex=%7B%5BU%2CX%5D%3DU%7D&bg=ffffff&fg=000000&s=0&c=20201002) , which implies that
, which implies that ![{[U_\alpha,X]=(\frac{X\alpha}{\alpha}-1)U_\alpha}](https://s0.wp.com/latex.php?latex=%7B%5BU_%5Calpha%2CX%5D%3D%28%5Cfrac%7BX%5Calpha%7D%7B%5Calpha%7D-1%29U_%5Calpha%7D&bg=ffffff&fg=000000&s=0&c=20201002) .
.
We see that we need compute integrals over sheared arcs 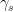 . Let
. Let 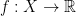 be a smooth function. Then
be a smooth function. Then
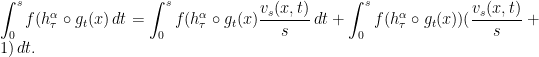
The second term is an ergodic integral which is easy to handle. The main term is the first term, which can be rewritten
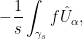
where  ,
,  .
.
To deduce mixing, one must estimate  inner products
inner products  . We integrate by parts
. We integrate by parts


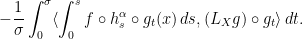
The second term is again an ergodic integral that tends to  .
.
5.3. Quantitative equidistribution estimates
We are interested in ergodic integrals of the form
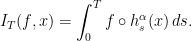
Flaminio-Forni treat the un-time-changed case 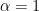 and show that
and show that

for some 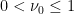 . One can adapt their arguments, using estimates by Bufetov-Forni, to the time-changed case, and get similar estimates.
. One can adapt their arguments, using estimates by Bufetov-Forni, to the time-changed case, and get similar estimates.
5.4. Additional references
Marcus original technique already proved mixing. His setting was Anosov flows, with their stable and unstable foliations. From these, a flow 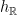 can be defined, which satisfies
can be defined, which satisfies

where $latex {s^*}&fg=000000$ has a continuous mixed partial second derivative $latex {\frac{\partial s^*}{\partial t \partial s}}&fg=000000$. So we see that time-changes were already in the picture.
Kushnirenko was able to prove mixing for smooth time-changes, assuming

Thus small time-changes are mixing. What about larger ones? This is still open.
Tiedra de Aldecoa uses a different method to prove absolute continuity of the spectrum for time changes satisfying (KC).
Generalizations. The setting is algebraic dynamics: a unipotent  -parameter subgroup acting on
-parameter subgroup acting on 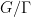 ,
,  semisimple Lie group.
semisimple Lie group.
Lucia Simonelli (Forni’s student) could prove absolute continuity of the spectrum for time changes satisfying (KC).
Davide Ravotti (my student) could prove quantitative mixing.
Kanigowski and Ravotti could prove quantitative 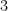 -mixing.
-mixing.
6. Heisenberg nilfows
Let  denote the Lie group of unipotent
denote the Lie group of unipotent  matrices, with
matrices, with  as standard generators of its Lie algebra,
as standard generators of its Lie algebra, ![{Z=[X,Y]}](https://s0.wp.com/latex.php?latex=%7BZ%3D%5BX%2CY%5D%7D&bg=ffffff&fg=000000&s=0&c=20201002) . Let
. Let 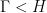 be a discrete cocompact lattice (for instance, unipotent matrices with integer entries). We call
be a discrete cocompact lattice (for instance, unipotent matrices with integer entries). We call  the Heisenberg nilmanifold.
the Heisenberg nilmanifold.  acts on
acts on  by right multiplication. The action of a
by right multiplication. The action of a  -parameter subgroup is called a nilflow.
-parameter subgroup is called a nilflow.
6.1. Classical results
Auslander-Green-Hahn (1963) studied unique ergodicity of nilflows. They showed that is 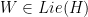 can be written
can be written 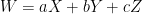 , for the nilflow defined by
, for the nilflow defined by 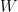 ,
,
unique ergodicity 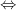 ergodicity
ergodicity 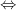 minimality
minimality 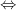
 and
and  are rationally independent.
are rationally independent.
Rational independence means that no linear relation with nonzero integral coefficients  can hold.
can hold.
In other words, if we project the situation to the  -torus
-torus 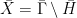 where
where ![{\bar H=H/[H,H]}](https://s0.wp.com/latex.php?latex=%7B%5Cbar+H%3DH%2F%5BH%2CH%5D%7D&bg=ffffff&fg=000000&s=0&c=20201002) ,
, 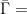 , then the flow
, then the flow 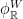 projects to a flow
projects to a flow  on
on 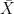 , and
, and
unique ergodicity for 
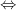 unique ergodicity for
unique ergodicity for 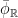 .
.
Here, we have used a theorem of Furstenberg on skew-products of rotations of the circle.
Definition 8 For real numbers 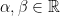 , let
, let  be the diffeomorphism of the
be the diffeomorphism of the  -torus defined by
-torus defined by
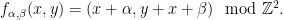
In general, a skew-product over a map  is a map
is a map 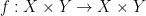 which is fiber-preserving (with respect to the projection
which is fiber-preserving (with respect to the projection  ) and the permutation of fibers is given by
) and the permutation of fibers is given by 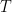 . In the example at hand,
. In the example at hand,  is isometric on fibers.
is isometric on fibers.
6.2. First return map
Lemma 9 Assume that the Heisenberg nilflow 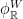 is uniquely ergodic. There is a transverse submanifold
is uniquely ergodic. There is a transverse submanifold 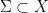 , diffeomorphic to a torus, such that the Poincaré return map
, diffeomorphic to a torus, such that the Poincaré return map  , given by
, given by 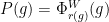 ,
,  the first return time to
the first return time to  , is one of the Furstenberg skew-products
, is one of the Furstenberg skew-products  .
.
Proof of the Lemma.  lifts to a vertical plane
lifts to a vertical plane 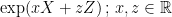 in
in  . Since
. Since  and
and 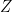 commute,
commute,  is diffeomorphic to a torus. If
is diffeomorphic to a torus. If 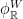 is uniquely ergodic,
is uniquely ergodic,  , so
, so  is transverse to the flow.
is transverse to the flow.
We show that  is a return time. We use the fact that
is a return time. We use the fact that  . So using the Campbell-Hausdorff-Dynkin formula, we compute
. So using the Campbell-Hausdorff-Dynkin formula, we compute
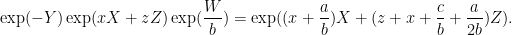
This point belongs to  , so
, so 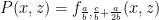 .
.
6.3. Lack of mixing
The above Lemma shows th at we can now focus on Furstenberg skew-products. We shall see that the parameter  plays no role, so we focus on
plays no role, so we focus on 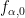 .
.
Definition 10 Given a map 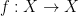 and a function
and a function 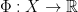 (called the roof function), we define the special flow
(called the roof function), we define the special flow  over
over  under
under 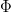 as follows: it is a flow on an
as follows: it is a flow on an  -bundle
-bundle  over the circle, the quotient of the vertical unit speed flow on
over the circle, the quotient of the vertical unit speed flow on 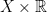 under the identification
under the identification
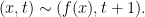
Fact. If a flow  admits a global Poincaré section
admits a global Poincaré section  with first return time
with first return time  , then
, then  is isomorphic to the special flow of the first return map with roof function
is isomorphic to the special flow of the first return map with roof function  .
.
In the case at hand, the roof function is constant. Therefore, the special flow is not mixing: if  ,
,  a short interval, so do its images by the vertical unit speed flow, and so do their projections to
a short interval, so do its images by the vertical unit speed flow, and so do their projections to  , which are the images of a set
, which are the images of a set  and its images by the special flow.
and its images by the special flow.
This shows that Heisenberg nilflows are never mixing.
7. Mixing time-changes
The following contents can be found in Avila-Forni-Ulcigrai. A recent generalization to all step 2 nilflows can be found in Avila-Forni-Ravotti-Ulcigrai. Ravotti has treated filiform nilflows.
Let  be a Heisenberg nilflow and
be a Heisenberg nilflow and 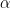 a smooth positive function on
a smooth positive function on  . Let
. Let
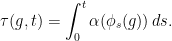
Then the time-change is given by
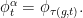
7.1. Time-changes versus special flows
We have seen that Heisenberg nilflows  are special flows with constant roof function. The time-change
are special flows with constant roof function. The time-change 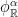 is again a special flow, over the same skew-product, but with roof function
is again a special flow, over the same skew-product, but with roof function
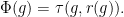
7.2. Trivial time-changes
Beware that there exist smooth time-changes which are trivial, i.e. smoothly conjugate to the original nilflow.
In general, adding a coboundary  to the roof function of a special flow produces an isomorphic flow. This leads us to the following problem: understand cohomology of nilflows. Here are our ultimate results.
to the roof function of a special flow produces an isomorphic flow. This leads us to the following problem: understand cohomology of nilflows. Here are our ultimate results.
Theorem 11 Let  be a There exists a dense set
be a There exists a dense set  in
in  of roof functions, and a vectorspace
of roof functions, and a vectorspace  of countable dimension and codimension, such that if a roof function
of countable dimension and codimension, such that if a roof function 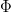 is chosen in
is chosen in 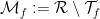 , the corresponding special flow
, the corresponding special flow  is mixing.
is mixing.
Moreover, for 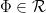 ,
,
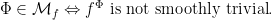
In fact, Katok has found a nice characterization of which  are smoothly trivial. I will come back to this next week. Today, I merely give one example.
are smoothly trivial. I will come back to this next week. Today, I merely give one example.
Example. 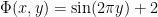 is a smoothly trivial roof function.
is a smoothly trivial roof function.
Under the assumption that 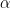 has bounded type, Kanigowski and Forni have proved quantitative mixing.
has bounded type, Kanigowski and Forni have proved quantitative mixing.
7.3. Idea of proof
We start from a Furstenberg skew-product 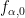 and play with roof functions. We use again mixing by shearing. We consider intervals in the fiber (i.e. in the
and play with roof functions. We use again mixing by shearing. We consider intervals in the fiber (i.e. in the 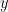 direction). We shall see that many of them shear in the flow (
direction). We shall see that many of them shear in the flow ( ) direction. But there are intervals which do not shear or shear in the other direction.
) direction. But there are intervals which do not shear or shear in the other direction.
7.4. Special flow dynamics
Given a point 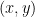 in the torus and
in the torus and 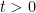 , we compute
, we compute  . When
. When  is large, we join bottom to roof several times. Let
is large, we join bottom to roof several times. Let

and
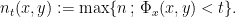
Then
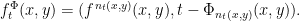
In order to exhibit shearing, we want to see how this changes in 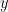 . Since
. Since  is an isometry in the
is an isometry in the 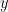 -direction, the
-direction, the 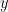 -derivative of the sum
-derivative of the sum 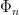 is the sum of
is the sum of 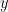 -derivatives, i.e.
-derivatives, i.e.
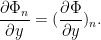
Take  trigonometric polynomials on the torus, which are positive.
trigonometric polynomials on the torus, which are positive.
Given 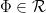 , let
, let 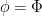 minus its average on the
minus its average on the 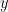 -fiber. Define
-fiber. Define 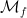 as the set of
as the set of 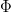 such that
such that  is not a measurable coboundary.
is not a measurable coboundary.
Step 1. Since  is not a measurable coboundary, the sums
is not a measurable coboundary, the sums  must grow,
must grow,

This relies on a result by Gottschalk-Hedlund, plus decoupling.
Step 2. The sums  are trigonometric polynomials of bounded degree. It follows that
are trigonometric polynomials of bounded degree. It follows that
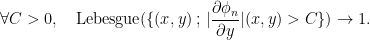
There can be flat intervals where no stretch occur, so one must throw them away. But the larger  , the shorter these intervals are.
, the shorter these intervals are.
Step 3. We use a polynomial bound on level sets of trigonometric polynomials.
The next class will deal with renormalization, deviations of ergodic averages. Only later shall we get back to Katok’s characterization of smoothly trivial special flows and to nilflows.
8. Short recap
Parabolicity is (a bit heuristically) defined by slow butterfly effect. This can be formalized for smooth flows, in terms of growth of derivatives under iteration. It is not that easy to build examples.
Presently, parabolic flows is the following list of examples,
- Horocycle flows of compact constant curvature surfaces.
- Unipotent flows (a generalization of the above).
- Nilflows and their time-changes.
- Smooth area-preserving flows.
- Linear flows on flat surfaces with conical singularites.
The two first are uniformly parabolic. The next is partially parabolic. The fourth is nonuniformly parabolic, the last is elliptic with singularities.
We have proven mixing via the technique of shearing.
Here is a further example where this technique works, due to B. Fayad, of an elliptic flavour. Start with a linear flow on the  -torus. If
-torus. If 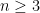 , Fayad has been able to construct anaytic time-changes which are mixing.
, Fayad has been able to construct anaytic time-changes which are mixing.
Such examples are very rare (they rely on parameters being very Liouville numbers).
9. Renormalization
The word here is taken in a meaning which differs from its use in holomorphic dynamics (not to speak of quantum mechanics).
The idea is to analyze systems which are approximately self-similar, and exhibit several time scales.
We introduce the renormalization flow  which rescales: long trajectories become short. Given a map
which rescales: long trajectories become short. Given a map 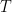 , one way to zoom in is to restrict
, one way to zoom in is to restrict 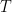 to a subspace
to a subspace  and replace
and replace 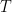 with the first return map to
with the first return map to  . Eventually rescale space afterwards. But there are other means.
. Eventually rescale space afterwards. But there are other means.
9.1. A series of examples
Example. Start with the horocycle flow 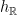 . The key relation is
. The key relation is

Applying the geodesic flow to a length  trajectory
trajectory  , we get a trajectory of length
, we get a trajectory of length  . So the geodesic flow achieves renormalization, on the same space, with no effort.
. So the geodesic flow achieves renormalization, on the same space, with no effort.
Example. The cat map  associated with the matrix
associated with the matrix  on the
on the  -torus. Let
-torus. Let  ,
,  denote the eigenvalues,
denote the eigenvalues,  the eigenvectors. Let
the eigenvectors. Let  be the linear flow in direction
be the linear flow in direction  , with unit speed. This is an elliptic flow.
, with unit speed. This is an elliptic flow.
Put 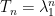 . Let
. Let
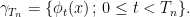
Put  . Then
. Then  maps
maps 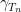 to a trajectory of
to a trajectory of  of length
of length  .
.
Express  as the composition of two Dehn twists,
as the composition of two Dehn twists,
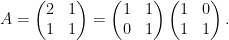
Rotate coordinates so that eigenvector  becomes vertical. Then
becomes vertical. Then  acts by a diagonal matrix in
acts by a diagonal matrix in  , which was denoted by
, which was denoted by  ,
,  earlier.
earlier.
Example. Higher genus toy model.
View a genus  surface
surface 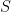 as a regular octagon in the Euclidean plane with edge identifications. Fix a direction
as a regular octagon in the Euclidean plane with edge identifications. Fix a direction 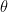 , consider the linear flow in direction
, consider the linear flow in direction 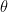 . It descends to a flow
. It descends to a flow  on the surface which is not well-defined at the vertex
on the surface which is not well-defined at the vertex
This generalizes to translation surfaces, made of a disjoint union of polygons, with identifications given by translations. The notion of a direction 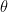 is well-defined on the surface (except at finally many singularities), whence a flow
is well-defined on the surface (except at finally many singularities), whence a flow  with singularities.
with singularities.
Consider matrix
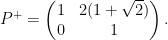
It applies a shear on the original regular octagon. Since 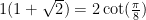 , This linear map induces a homeomorphism of the surface
, This linear map induces a homeomorphism of the surface 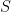 . Let us repeat in different direction (rotation by 45 degrees), get matrix
. Let us repeat in different direction (rotation by 45 degrees), get matrix  . Let
. Let  , this is a hyperbolic matrix with eigenvalues
, this is a hyperbolic matrix with eigenvalues 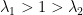 . We get again an affine automorphism
. We get again an affine automorphism  of the surface. Let
of the surface. Let  denote the linear flow in the direction of the eigenvector
denote the linear flow in the direction of the eigenvector  . Then
. Then
B. Veech has shown that the affine group of the surface is generated by  and the order
and the order 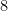 rotation. This is as large as the affine group of a translation surface can be. Therefore,
rotation. This is as large as the affine group of a translation surface can be. Therefore, 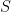 is clled the Veech surface.
is clled the Veech surface.
For almost every direction 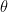 (the condition is that
(the condition is that  ), there exists a sequence of hyperbolic automorphisms
), there exists a sequence of hyperbolic automorphisms 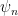 with unstable direction
with unstable direction 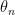 that converge to
that converge to 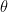 .
. 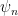 can be used to renormalize
can be used to renormalize  .
.
Example.
For a slightly deformed octagon  , the affine group of the corresponding surface
, the affine group of the corresponding surface 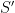 is trivial. Nevertheless, almost every linear flow
is trivial. Nevertheless, almost every linear flow  is still renormalizable: there is a sequence of surfaces
is still renormalizable: there is a sequence of surfaces  and affine hyperbolic morphisms
and affine hyperbolic morphisms  with expanding direction
with expanding direction 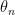 converging to
converging to 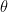 .
.
Indeed, consider the space 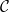 of linear flows on translation surfaces of genus
of linear flows on translation surfaces of genus  . The flow of diagonal matrices acts on this space, generating a flow
. The flow of diagonal matrices acts on this space, generating a flow 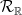 on
on 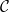 , known as the Teichmüller flow.
, known as the Teichmüller flow.
Theorem 12 (Masur-Veech) 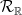 is recurrent.
is recurrent.
Therefore for almost every linear flow, there exists a sequance  such that
such that  tends to
tends to 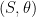 .
.
9.2. What is renormalization good for?
It is used to put diophantine conditions on linear flows in higher genus. See my ICM 2022 talk (watch it on line on july 11th). I do not pursue this topic further.
It is used to study deviations of ergodic averages. Assume a flow  is uniquely ergodic. Ergodic integrals take the form
is uniquely ergodic. Ergodic integrals take the form
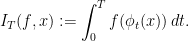
By the ergodic theorem, 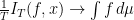 for every
for every  . Let us focus on our favourite example, the Veech surface. The invariant measure is area in the plane. Unique ergodicity is a theorem of Masur, Kerckhoff-Masur-Smillie
. Let us focus on our favourite example, the Veech surface. The invariant measure is area in the plane. Unique ergodicity is a theorem of Masur, Kerckhoff-Masur-Smillie
We show that for functions with vanishing average,
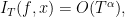
for some 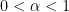 (discovered by Zorich, conjectured by Kontsevitch-Zorich, proven by Forni).
(discovered by Zorich, conjectured by Kontsevitch-Zorich, proven by Forni).
Fix a basis  of
of  . Fix a section
. Fix a section  of the linear flow. Take trajectories
of the linear flow. Take trajectories 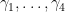 from
from  and to their first return to
and to their first return to  . Closing them by segments of sigma gives representatives of the basis of homology. Let
. Closing them by segments of sigma gives representatives of the basis of homology. Let
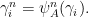
Let  be the matrix expressing the homology basis
be the matrix expressing the homology basis 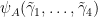 is the initial basis. Let
is the initial basis. Let  denote the eigenvalues of
denote the eigenvalues of 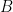 . By continuity of
. By continuity of  , up to a small error,
, up to a small error,
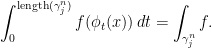
Let us start from a large 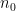 instead of
instead of  ,
,
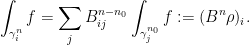
For simplicity, let us assume that 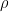 is the eigenvector
is the eigenvector  . Then
. Then
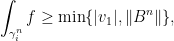
a contradiction. Therefore 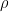 must be a combination of
must be a combination of 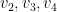 . Thus
. Thus

On the other hand, 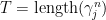 grows like
grows like 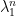 . Let
. Let 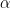 satisfy
satisfy
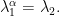
Then 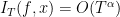 , as announced.
, as announced.
Of course, I cheated a bit, some more regularity of  is needed.
is needed.
In that example, the fact that  can be explicitly. Tomorrow, I will mention results on this for general translation surfaces.
can be explicitly. Tomorrow, I will mention results on this for general translation surfaces.
And then, back to nilflows.
9.3. Area preserving flows on surfaces
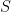 compact connected oriented surface of genus
compact connected oriented surface of genus  . Let
. Let  be a flow which preserves a smooth measure
be a flow which preserves a smooth measure  . Note that
. Note that  has fixed points, which are all of saddle type. In the next theorem, the number and types of fixed points are fixed.
has fixed points, which are all of saddle type. In the next theorem, the number and types of fixed points are fixed.
Theorem 13 (Zorich, Forni, Avila-Viana) There exists 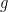 distinct positive exponents
distinct positive exponents  such that almost every choice of
such that almost every choice of  (Katok’s fundamental class, described in terms of periods) is uniquely ergodic, and for all smooth functions
(Katok’s fundamental class, described in terms of periods) is uniquely ergodic, and for all smooth functions  ,
,
 for all
for all  .
.
In this statement,  means a function
means a function  such that
such that
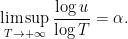
Furthermore,  is a distribution on
is a distribution on 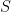 , and
, and 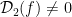 .
.
This type of behavior is called a power deviation spectrum. It was conjectured by Kontsevitch and Zorich, then proved by Zorich in 1997 for special functions  , with only one term
, with only one term  . Then Forni obtained a proof in 2002 for functions with support away from fixed points, up to distinctness of exponents which was proven by Avila-Viana. Bufetov gave a more precise version, transforming the result into an asymptotic expansion. With Fraczek, we gave a different proof based on Marmi-Moussa-Yoccoz). Finally, Fraczek-Kim could handle generic saddles. The expansion then involves extra terms depending on saddles (and not on
. Then Forni obtained a proof in 2002 for functions with support away from fixed points, up to distinctness of exponents which was proven by Avila-Viana. Bufetov gave a more precise version, transforming the result into an asymptotic expansion. With Fraczek, we gave a different proof based on Marmi-Moussa-Yoccoz). Finally, Fraczek-Kim could handle generic saddles. The expansion then involves extra terms depending on saddles (and not on  ).
).
9.4. Idea of proof
Choose coordinates such that  appears as a time-change of a linear flow on a translation surface. The time change is smooth only away from fixed points. If function
appears as a time-change of a linear flow on a translation surface. The time change is smooth only away from fixed points. If function  has support away from fixed points, one is reduced to study deviation for linear flows. For a general linear flow, a renormalization is given by a sequence of matrices
has support away from fixed points, one is reduced to study deviation for linear flows. For a general linear flow, a renormalization is given by a sequence of matrices  , the Kontsevitch-Zorich cocycle. Eigenvalues are replaced with ratios of Lyapunov exponents.
, the Kontsevitch-Zorich cocycle. Eigenvalues are replaced with ratios of Lyapunov exponents.
10. More on renormalization
10.1. Back to nilflows
Let 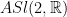 denote the stabilizer of vector
denote the stabilizer of vector  is
is 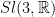 . Defined
. Defined  by
by
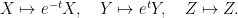
Then  is recurrent, its has been used as a renormalization by Flaminio-Forni, in order to prove polynomial deviations of ergodic averages.
is recurrent, its has been used as a renormalization by Flaminio-Forni, in order to prove polynomial deviations of ergodic averages.
10.2. Renormalizable parabolic flows
Horocycle flows: yes.
Unipotent flows: unknown.
Heisenberg nilflows: yes.
Higher step nilflows: unknown in general. Special case (special flows over skew products) studied by Flaminio-Forni. In that case, the maps  diverge.
diverge.
Linear flows over higher genus surfaces (they are smooth and area-preserving).
More general smooth flows on surfaces (not necessarily area preserving). Then  typically diverges. This is related to generalized interval exchange transformations.
typically diverges. This is related to generalized interval exchange transformations.
11. Isomorphisms between time-changes
Recall that a time-change 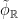 of a flow
of a flow  is
is
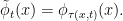
When do such a change lead to a genuinely different, nonisomorphic flow?
11.1. Setting of special flows
Let  be a map. Given a roof function $latex {\Phi:Y\rightarrow{\mathbb R}_{>0}}&fg=000000$, the flow of translations on
be a map. Given a roof function $latex {\Phi:Y\rightarrow{\mathbb R}_{>0}}&fg=000000$, the flow of translations on 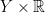 descends to a flow $latex {\psi^{f,\Phi}_{\mathbb R}}&fg=000000$ on the quotient space
descends to a flow $latex {\psi^{f,\Phi}_{\mathbb R}}&fg=000000$ on the quotient space
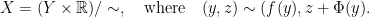
Lemma 14 Let 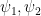 be special flows over the same map
be special flows over the same map  , under roofs
, under roofs  and
and  . If there exists a function
. If there exists a function 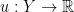 such that
such that
 then the two special flows are isomorphic.
then the two special flows are isomorphic.
Indeed, look for a conjugating homeomorphism of the form
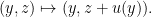
It commutes with translations and maps one equivalence relation to the other.
11.2. Cohomological equations
The operator 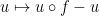 is called the coboundary operator. Hence the equation
is called the coboundary operator. Hence the equation 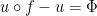 with unknown
with unknown  is called a cohomological equation. It sometimes appears with a twist:
is called a cohomological equation. It sometimes appears with a twist: 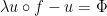 , for some
, for some  .
.
There are obvious obstructions.
If  is a periodic point, i.e.
is a periodic point, i.e.  , then
, then

So every periodic orbit gives an obstruction. If  is hyperbolic, it is essentially the only one.
is hyperbolic, it is essentially the only one.
More generally, if  is an invariant measure, then
is an invariant measure, then
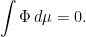
So every invariant measure gives an obstruction.
11.3. An elliptic example
If  is elliptic, this is sometimes sufficient, e.g. for circle rotations
is elliptic, this is sometimes sufficient, e.g. for circle rotations 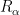 : if
: if 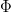 is a trigonometric polynomial and
is a trigonometric polynomial and  , then there exists a solution
, then there exists a solution  . If
. If 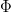 is smooth, a Diophantine condition on
is smooth, a Diophantine condition on 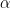 is required in addition for the solution
is required in addition for the solution  to be smooth. Indeed, in Fourier, if
to be smooth. Indeed, in Fourier, if
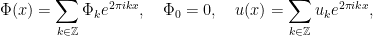
then
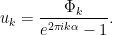
For 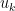 to decay superpolynomially, one needs that
to decay superpolynomially, one needs that
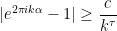
for some  , which amounts to
, which amounts to 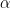 being badly approximable by rationals.
being badly approximable by rationals.
11.4. Parabolic case
In the parabolic world, in addition to invariant measures, invariant distributions provide further obstructions.
Remember that Heisenberg nilflows are special flows with constant roof over Furstenberg skew-products of the form
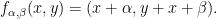
We need study the corresponding cohomological equation.
Proposition 15 In Fourier series, if
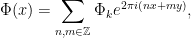 then the cohomological equation has a formal solution if and only if all
then the cohomological equation has a formal solution if and only if all 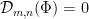 where
where  is the distribution such that
is the distribution such that 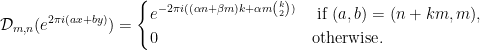
Indeed, let 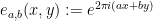 , and
, and
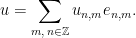
Then
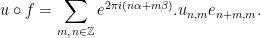
The matrix  acts on
acts on 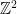 . It has a family of orbits
. It has a family of orbits  and
and  orbits
orbits
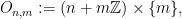
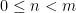 , for each
, for each 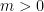 .
.  splits accordingly, so the cohomological equation can be solved independently in each summand
splits accordingly, so the cohomological equation can be solved independently in each summand

and on
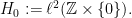
For 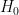 ,
,  acts like the circle rotation
acts like the circle rotation 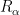 , so we already understand the necessary condition, given by invariant measures.
, so we already understand the necessary condition, given by invariant measures.
For fixed 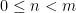 and
and 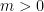 , let us denote
, let us denote
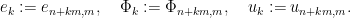
The cohomological equation reads

where
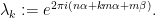
Recursively, one gets
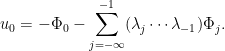
Then
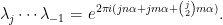
Similarly, the equation
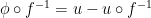
yields
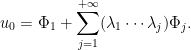
Combining both leads to
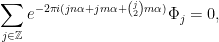
i.e.  .
.
Conversely, one can see that this conditions are sufficient for existence of a formal solution, and for existence of smooth solutions under Diophantine conditions.
11.5. More general results on cohomological equations in parabolic dynamics
- The case of Heisenberg nilflows, which we just treated, is due to Katok.
- More general nilflows have been studied by Flaminio-Forni, as well as horocycle flows.
- Linear flows on higher genus surfaces are due to Forni. In this case, one gets
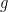 distributions
distributions 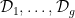 . If a smooth function
. If a smooth function  is killed by all of them, ergodic integrals stay bounded. This is equivalent to
is killed by all of them, ergodic integrals stay bounded. This is equivalent to  being a coboundary, according to Gottschalk-Hedlund.
being a coboundary, according to Gottschalk-Hedlund.
11.6. Cocycle effectiveness
Sometimes, the cohomological equation with measurable data is needed. It is much more difficult, but some miracle occurs in the parabolic setting.
Definition 16 Given a map 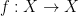 , say a function
, say a function 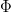 on
on  is a measurable coboundary of the exists a measurable
is a measurable coboundary of the exists a measurable  such that
such that 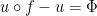 .
.
The theorem on mixing smooth time-changes of Heisenberg nilflows (Avila-Forni-Ulcigrai) required the roof not to be a measurable coboundary. It turns out that here, this is equivalent to not being a smooth coboundary.
Proposition 17 Let us study the Furstenberg skew-product  on the
on the  -torus. Let
-torus. Let 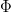 be a smooth function on
be a smooth function on  . Then
. Then
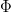 is not a smooth coboundary
is not a smooth coboundary 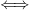
 is not a measurable coboundary.
is not a measurable coboundary.
Indeed, let
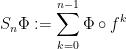
denote the Birkhoff sums. Then Flaminio-Forni establish quadratic upper bounds: there exists a sequence  such that
such that

Matching lower bounds exist: if 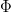 is not a smooth coboundary, there exists a nonvanishing
is not a smooth coboundary, there exists a nonvanishing  , and
, and

If 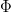 is a measurable coboundary,
is a measurable coboundary,  , then
, then

stays bounded on a set of almost full measure, and grows at most quadratically on the complement. This contradicts the quadratic lower bound.
11.7. More general nilflows
Theorem 18 (Avila-Forni-Ravotti-Ulcigrai) For general nilflows of step  , there exists a dense (in
, there exists a dense (in 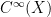 ) class
) class  of generators
of generators 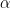 of time-changes, which are
of time-changes, which are
- either measurably trivial (i.e. measurably conjugate to the nilflow);
- or mixing.
Unfortunately, the set  is not explicitly describable like in the Heisenberg case.
is not explicitly describable like in the Heisenberg case.
The proof is an induction on central extensions. It uses mixing by shearing.
12. More examples of parabolic dynamics
12.1. Parabolic perturbations which are not time-changes
These were discovered by Ravotti during his PhD at Princeton.
Here, parabolic means that the derivative of the flow grows polynomially. It implies that smooth time-changes are still parabolic.
Start with 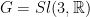 ,
,  a cocompact lattice,
a cocompact lattice,  . Let
. Let 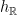 be the flow generated by a unipotent element
be the flow generated by a unipotent element 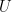 . Let
. Let 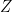 belong to the center of the minimal unipotent and
belong to the center of the minimal unipotent and 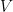 such that
such that ![{[U,V]=-cZ}](https://s0.wp.com/latex.php?latex=%7B%5BU%2CV%5D%3D-cZ%7D&bg=ffffff&fg=000000&s=0&c=20201002) .
.
Let 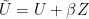 where
where  is a function on
is a function on  such that
such that  . Let
. Let 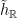 denote the corresponding flow.
denote the corresponding flow.
Theorem 19 If 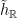 preserves a smooth measure
preserves a smooth measure 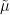 with
with 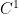 density, then
density, then 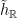 is
is
- parabolic:
 ;
; - ergodic;
- mixing.
Remark. Existence of 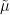
 there exists a time-change of the
there exists a time-change of the 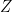 -flow which commutes with
-flow which commutes with 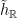 .
.
Remark. There exists such 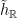 which are not smoothly isomorphic to
which are not smoothly isomorphic to 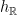 . This follows from the failure of cocycle rigidity for parabolic actions, due to Wang, following many people.
. This follows from the failure of cocycle rigidity for parabolic actions, due to Wang, following many people.
Remark. Ergodicity needs be proven, it does not follow from general principles.
The proof relies on mixing by shearing, although in a setting different from what we have already met. Consider arcs of orbits of 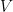 and push them by
and push them by 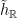 . Since
. Since
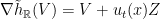
where 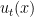 is an ergodic integral for
is an ergodic integral for 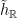 .
.
12.2. What else can shearing be used for?
A strong, quantitative, shearing can be used to establish spectral results. Here, I mean the spectrum of the Koopman operator
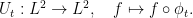
To each  , there corresponds a spectral measure
, there corresponds a spectral measure  on
on 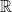 . Its Fourier coefficients are given by selfcorrelations of
. Its Fourier coefficients are given by selfcorrelations of  , i.e.
, i.e.
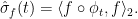
The spectrum of 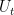 is absolutely continuous
is absolutely continuous 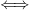 for all
for all  ,
, 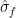 is absolutely continuous
is absolutely continuous 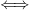 for all
for all  ,
,  .
.
If  is ergodic, it is enough to study functions
is ergodic, it is enough to study functions  which are smooth coboundaries.
which are smooth coboundaries.
Theorem 20 (Forni-Ulcigrai) Smooth time-changes of a horocycle flow has absolutely continuous spectrum.
The proof uses quantitative bounds

Since 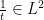 , this implies absolute continuity.
, this implies absolute continuity.
Fayad-Forni-Kanigowski consider smooth area-preserving flows on the  -torus with a stopping point.
-torus with a stopping point.
12.3. Ratner property
M. Ratner uses a quantitative form of shearing for unipotent flows.
Shearing takes some time. Ratner requires the following:
For all  , for all large enough
, for all large enough 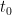 , there exists a set
, there exists a set 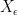 of measure
of measure  , for all pairs
, for all pairs  not in the same orbit, but such that
not in the same orbit, but such that 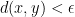 , there exists
, there exists 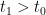 such that
such that
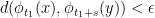
and
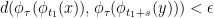
for all ![{\tau\in [t_1,(1+K)t_1]}](https://s0.wp.com/latex.php?latex=%7B%5Ctau%5Cin+%5Bt_1%2C%281%2BK%29t_1%5D%7D&bg=ffffff&fg=000000&s=0&c=20201002) .
.
For a long time, this was used only in algebraic dynamics, until a more flexible variant, called switchability, was introduced. It means that one can switch past and future.
Fayad-Kanigowski and Kanigowski-Kulaga-Ulcigrai established this variant for typical smooth area-preserving flows on surfaces. This implies mixing of all orders.
Here is another application if these ideas:
Theorem 21 (Kanigowski-Lemanczyk-Ulcigrai) For all smooth time-changes 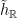 of the horocycle flow, the rescaled flow
of the horocycle flow, the rescaled flow  is not isomorphic to
is not isomorphic to 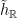 .
.
They are actually disjoint in Furstenberg’s sense. Recall that the horocycle flow itself is isomorphic to its rescalings.
We use a disjointness criterion based on this switchable variant of Ratner’s property.
12.4. Summary
- Parabolic means slow butterfly effect.
- Typically slow mixing.
- Slow equidistribution.
- Disjointness of rescalings.
- Obstructions to cohomological equation.
Tools:
- Shearing.
- Ratner property and switchability.
- Renormalization.
a positive integer,
an integer. There exist infinitely many prime numbers
such that
if and only if
.
.
of primes are evenly distributed.
is a Dirichlet character mod
if
for all
,
for all
,
.
-function
, the trivial character, then
is entire. Dirichlet’s theorem follows from
. The prime number theorem on arithmetic progressions follows from
for all
and on a neighborhood of that line. Improving the remainder to be polynomial of degree
is
amounts to nonvanishing of
on
. This is hard. The special case
is known as Generalized Riemann Hypothesis.
has a probability to be prime which is
, the expected error from this random model is
.
-functions are statistical: what happens on the average over
?
,
or
,
.
denote a field with
elements,
denotes the ring of polynomials with coefficients in
. I intend to replace integers with
. For instance, the Euclidean algorithm works on polynomials.
the set of monic polynomials (thought of as an analogue of positive integers).
, its absolute value is
, which is equal to the cardinality of the quotient ring
.
is a Dirichlet character mod
if
for all
,
for all
,
.
-function is
.
is entire for
, because it is a polynomial in
. Indeed,
vanishes for
, since each residue class mod
occurs
times and, by orthogonality of characters,
.
for
. If we can improve nonvanishing we get information on the number of irreducible
such that
and
.
for
.
. Katz, Katz-Sarnak, Deligne answered statistical questions in the limit where
tends to infinity.
is primitive if there is no character
of smaller modulus
such that
for all
with
.
is odd if
for some
.
is primitive and odd. Then
is a polynomial in
of degree exactly
with all roots on
. It follows that
is the characteristic polynomial of
where
is a unitary matrix.
is squarefree and
, there a exists a map
equidistribute in the unitary group as
tends to infinity. It kills hope to control its nonvanishing simultaneously for all
.
be squarefree of degree
. Then
.
is large enough.
. Since the middle of XXth century, one knows that such a counting can follow from the same topological techniques used to describe solutions over the complex numbers.
,
involving the number of
such that
and
.
-tuples of natural numbers summing to
of sets, each of which is the set of solutions to
equations in
variables, over
(the unknowns are the coefficients of the monic polynomials
).
be a scheme of finite type over
. Then the number of points of
over
is
on
are algebraic integers of absolue value
.
times the dimension of cohomology. This allows to conclude
be a monic squarefree polynomial of degree
over a field
. Let
be prime to
. Consider the union of the schemes parametrizing the set of
whose product equals
. Then the cohomology of the points over
splits as the sum of a boring piece (independent on
) and an interesting piece which is
-dimensional if
, and otherwise its dimension is at most
